The aerodynamics behind the Cristiano Ronaldo free kick with CFD simulation
2018 FIFA World Cup. 32 teams battling it out in the most popular event in sports history.
POR 2 | ESP 3 – 87:01
Two of the favorites to lift the cup. Portugal is down a goal desperate to salvage a point. Millions of eyeballs are glued to the game. Gerald Pique of Spain fouls the most famous player on the planet (Sorry, Lionel) just outside the box. Free kick for Portugal. 22 meters away from the goal. Enter Cristiano Ronaldo.
“Moments maketh the man” – A perfectly executed free kick with dip and curve beats the defenders wall and the Spanish goalkeeper’s outstretched hands. In just 0.82 seconds, Ronaldo waltzed, nah – he swerved right into the pantheon of World Cup legends. The magnificent free kick and the point it earned for Portugal made all the difference in a group that was decided in the last minute of the last game, thanks to the tenacious displays of Iran and Morocco.
If Portugal goes on to win it all, this curling thunderbolt from Ronaldo will forever be remembered as the moment ‘A Seleção das Quinas’ started their march towards glory. If they don’t, it will still be one of the great world cup moments, thanks to the sheer genius of Ronaldo and a certain ‘Gustav Magnus’. Magnus, who?
Ronaldo Free Kick Magic
Before I go further, let’s watch the magic once more. Go to 0:29 seconds. Watch it. Rub your eyes. Watch it again.
(Courtesy: FOX Soccer)
Notice the ‘banana’ curve of the ball? To beat David De Gea, one of the top goalkeepers in the world, Ronaldo had to get the ball up and over the wall of defenders from just 22 meters away at just the right height and speed. But that wasn’t enough.
Ronaldo hits the ball slightly off center to the right away from the goal, imparting a spin on the ball causing it to curve back into the goal. It was this ‘banana swing’ that made the goal possible, leaving the fleet-footed De Gea rooted to his spot. To execute this perfectly under pressure on the biggest stage in sports takes hours of practice and skill and genius. And the Magnus effect.

Soccer balls and the Magnus effect
“Magnus Effect” is the curving of a spinning spherical object to one side because of the uneven air flow generated around the object from its spin.
A spinning soccer ball moving through the air is subject to different forces that determine the ball’s trajectory. The drag force results from the friction of air molecules on the surface (friction drag) and the pressure imbalance due to the airflow around the ball (pressure drag). Drag slows the ball down – a universal aerodynamic phenomenon, be it airplanes, cars or sports balls. Lift force creates a lift on the object due to pressure difference (this is why planes fly). Then there’s the side force – this is where Magnus effect comes in.
At low speeds, flow around the ball is laminar (a uniform flow of air layers that are undisturbed). The boundary layer, the thin layer of flow close to the surface, separates away from the ball immediately after the center leaving a region of chaotic, disturbed flow behind the ball. At high speeds, flow around the ball is turbulent including in the boundary layer. The turbulence excites the molecules of air and causes them to stick closer to the surface longer. As a result, the boundary layer separates farther towards the back of the ball, resulting in a smaller wake. So far so good? See the picture below to understand this better.
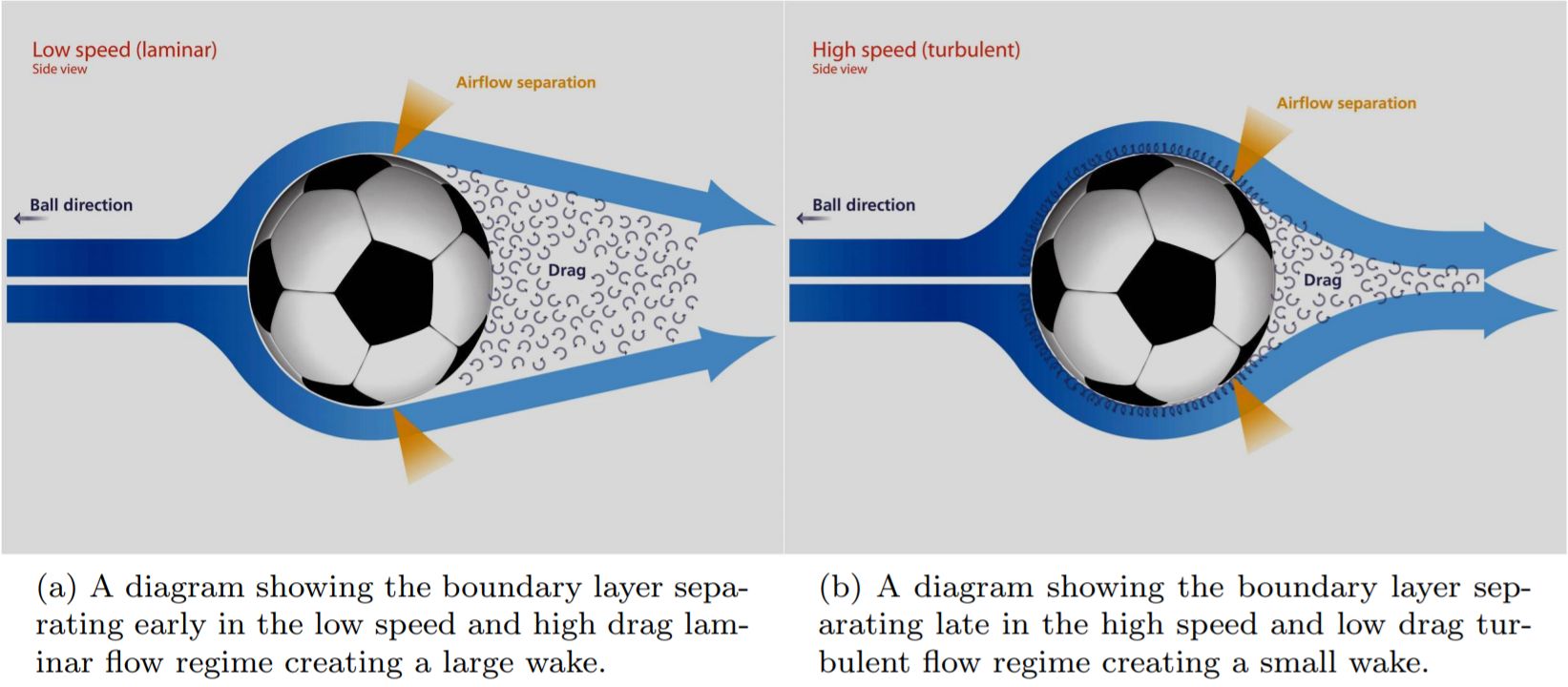
As the ball rotates in a free kick, the boundary layer close to the surface is pulled in the direction of rotation, leading to delayed separation on this side. On the other side, the rotation leads to earlier separation. This results in a force that pushes the ball sideways towards the area of lower pressure (remember high school physics? Air flows from high pressure to low pressure) – the Magnus Force.

All this is complicated by the roughness and seams on the ball. There’s been enough research on the aerodynamics of different balls in wind tunnels. The consensus is that the number of panels on the ball, their shape, the seam depth and height are all major factors in the ball’s behavior. World cup balls don’t have the traditional 32 panels anymore, with Adidas continuously innovating with ball design.
Adidas Telstar 18 – Ronaldo’s friend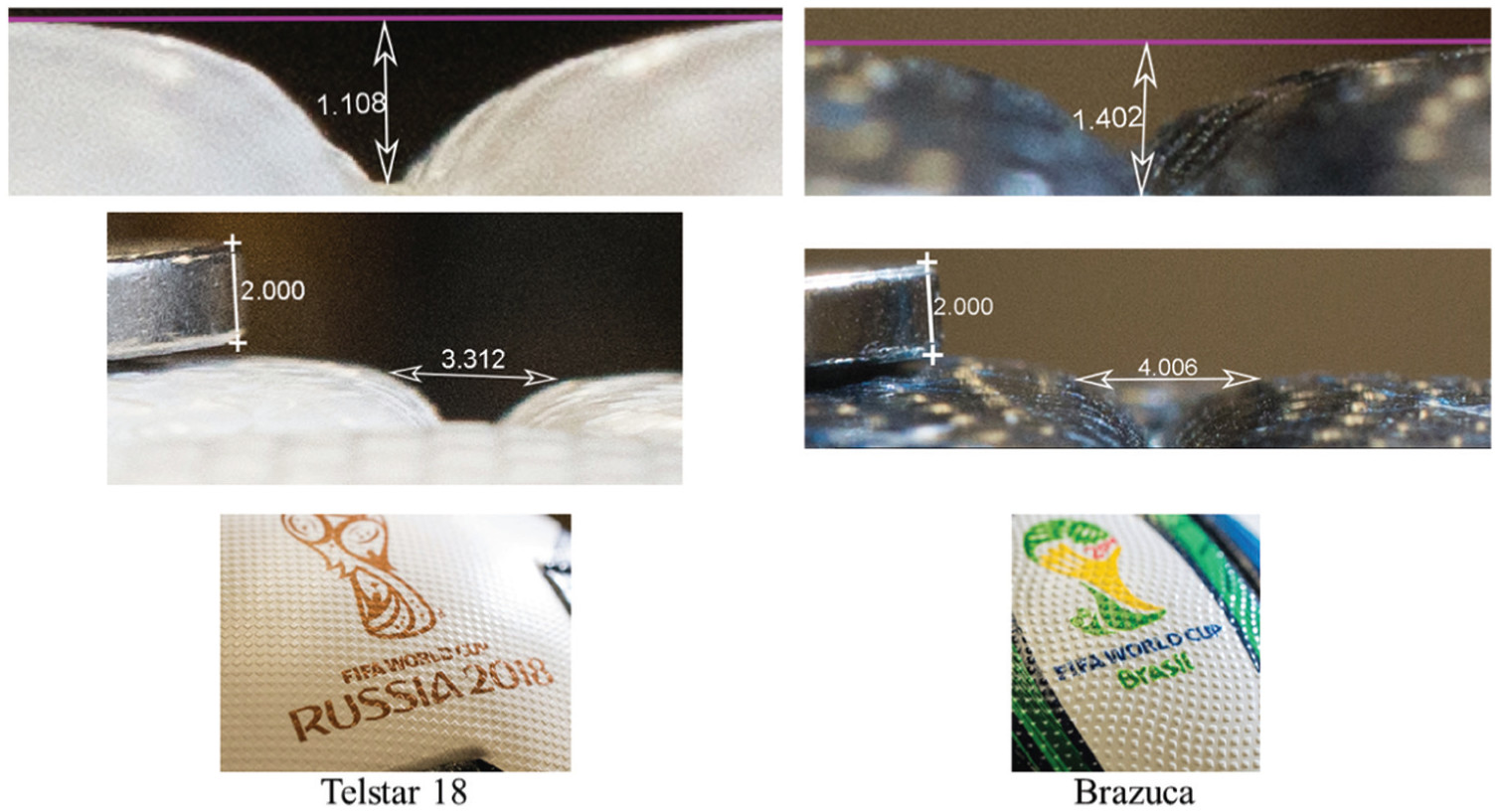

(Courtesy: Aerodynamic and surface comparisons between Telstar 18 and Brazuca, John Eric Goff, Sungchan Hong, and Takeshi Asai, Proceedings of the Institution of Mechanical Engineers, Part P: Journal of Sports Engineering and Technology)
The Telstar 18 is the official ball of the 2018 World Cup. John Eric Goff and co. have already put the ball through wind tunnel tests and compared the aerodynamic and surface properties with the Brazuca from 2014. The image above show the difference in panel shapes, surface texture and seam depths. It’s a testament to Adidas’ engineering that even with these changes, the balls are aerodynamically stable and behave similarly.
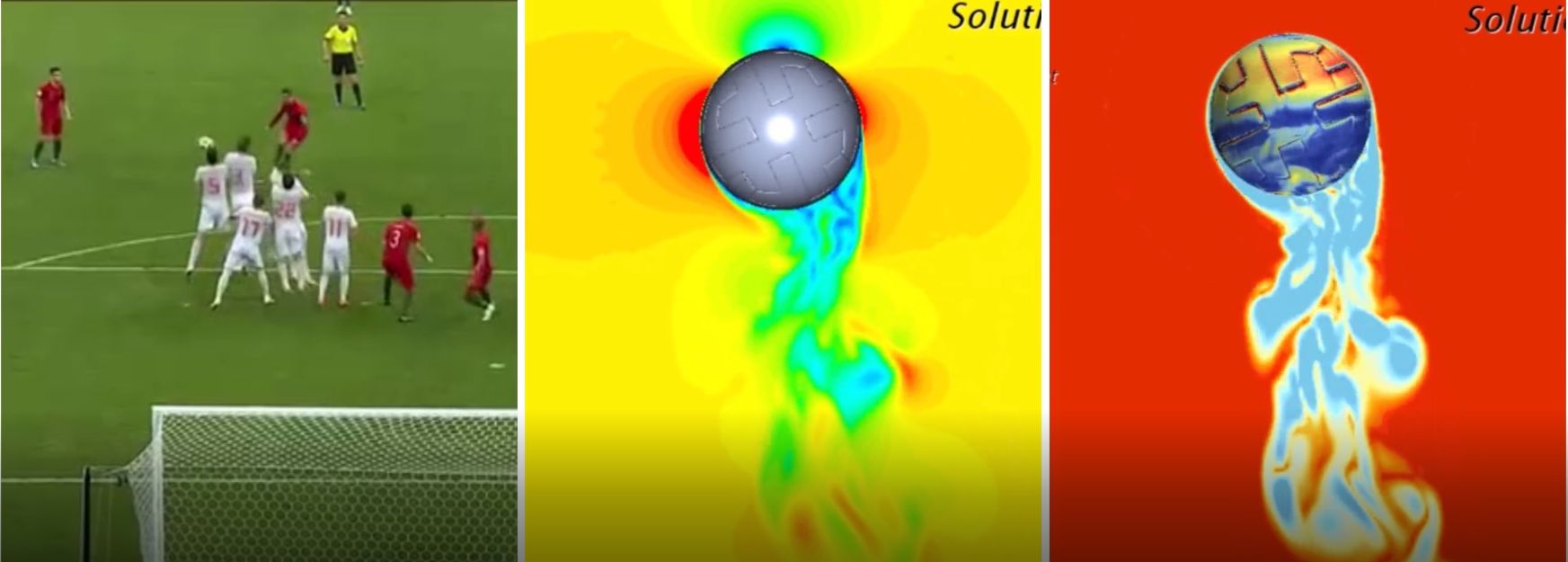
CFD simulation of Ronaldo’s free kick
To understand the exact aerodynamics behind the now-famous free kick, I decided to simulate the kick in a “virtual wind tunnel” using Simcenter STAR-CCM+, the computational fluid dynamics (CFD) software from Siemens PLM Software. CFD is a fluid mechanics sub-segment that uses mathematics, physics and software to understand the behavior of fluids. Used universally in the design of most modern day products, be it aircraft, ships, oil platforms, cars or medical devices, Simcenter STAR-CCM+ enables the creation of a ‘digital twin’ of the soccer ball that accurately represents the ball behavior in real life conditions.
To perform the simulation, a 3D model of the ball was used. The kick was taken from around 22 meters from the goal and took 0.82 seconds to find the back of the net. The ball weighs 0.436 kg with an approximate spin of around 5 revolutions per second. The ball traveled at around 26.8 m/s (60 mph) from Ronaldo’s foot. The ground atmospheric conditions were assumed to be a regular day at sea level. Predicting boundary layer transition is key in soccer ball aerodynamics and a special Gamma Re Theta transition model in STAR-CCM+ was used to predict this.
Simulation always needs to be validated against test data. To validate the CFD model, the force coefficients were compared against wind tunnel data from Goff and co for a non-spinning Telstar 18. The drag coefficient results compared against data in the free kick regime are in the table below.
| CD (Wind tunnel) | CD (STAR-CCM+) | |
| 69 mph | 0.201 | 0.2209 |
| 76 mph | 0.196 | 0.222 |
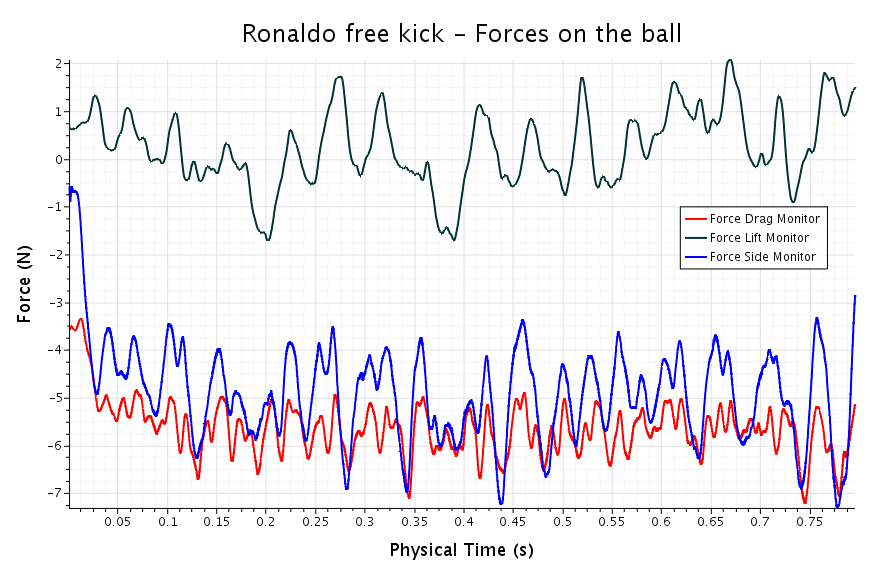
Accounting for unknown data and assumptions, the drag, lift and side forces matched reasonably well with test data. Flow physics were also investigated to validate the simulation model.
The forces on the spinning ball from STAR-CCM+ were averaged out over the time in flight. Notice the side force is hovering around 5 Newton on the negative side? There’s your curve. This force acting continuously on the ball is what curves it in flight.
Lift force – 0.277N; Drag force – 5.645 N; Side force (Magnus) – 4.9N.
From these, the deviation of the ball from a straight path can be calculated with simple algebra (See Figure for clarity). Final deviation for Ronaldo’s kick with Telstar 18 from STAR-CCM+ = 3.9 meters
The Perfect Curve
Considering the short distance and the fact that Ronaldo kicked the ball with spin away from the post before it curves in, two things are clear:
- He is at the peak of his powers to execute such a perfect curve kick
- He could do so because Adidas delivered a ball with better aerodynamics and more predictable behavior
You can see the velocity and vorticity (a measure of rotation in the fluid) behind the ball during the kick. The net side force is a result of the vortex pairs in the wake and the size of the vortices. Though the wake skews to one side causing the side force, the fluctuations in the wake are small for the spinning ball giving rise to the smoother curve. This is why players trust the ‘curve’ and kick it outside the post trusting Magnus and the aerodynamics to curve it into the goal.
The simulation results were generated by splitting the ball and the surrounding area into 30 million hexahedral cells and solving Navier-Stokes equations (these govern flow physics) on these cells. How complex is this? One simulation took 24 hours to run on 96 computing cores. Physics quantities were computed at every 0.0005 seconds, ensuring that the flow fluctuations in time and space were accurately captured. The ball was rotated by 1 degree for every 0.0005 seconds.
The initial seam orientation plays a role in the side force generated. So does the surface texture of the ball (a measure of how rough the ball is). Also crucial is the exact spin rate of the ball in flight. Due to lack of information on these, assumptions were made. A surface roughness of 5 microns was applied uniformly on the ball. The ball geometry is a representation of the actual ball. A laser scan of the ball would be more accurate. The spin rate was approximated visually. The arc of the ball from the ground to its position above the wall and the resulting dip was not accounted for either. Knowing these would result in an even better prediction.
Did the Telstar 18 help? Yes of course.
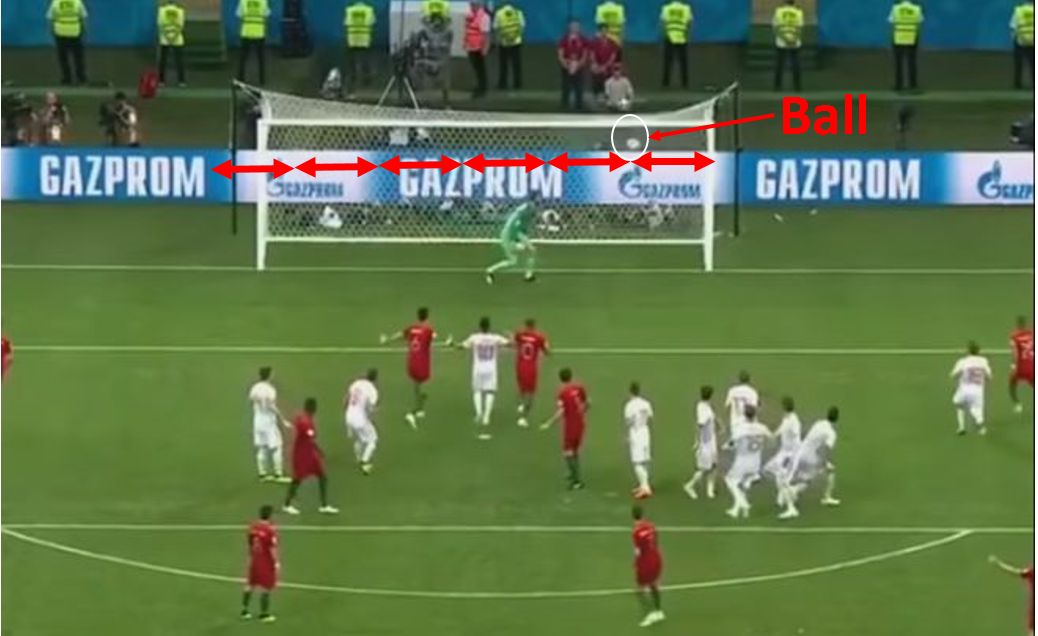
Studies from John Eric Goff have already shown that the Telstar 18 and Brazuca are aerodynamically similar and are much more predictable in their behavior. Did this help this specific kick? I think so. De Gea is left stranded in his spot mainly because the ball curves outside the goalpost before curving back in. The goalpost is 7.32 m wide. A fair estimate of the actual sideways deflection of the ball is around 3.5 meters. Simulations predicted 3.9 m, taking into account the assumptions made and the lack of data and scanned model. What I was more interested in is to understand just how much influence the ball can have in changing the course of a match and with it, history.
I repeated the same simulation with the 2010 Jabulani ball and got the following results.
Lift force – 0.3667 N; Drag force – 5.338 N; Side force (Magnus) – 5.647 N.
Final deviation for the Ronaldo free kick from STAR-CCM+ = 4.6 meters
De Gea gave up on stopping the kick the minute he realized where the ball was headed – towards the corner and out of his reach. He is 6 ft 3 in (1.9 m) tall. His overall reach is around 2.2 m when diving. De Gea’s starting position was around 2.75 m from the left post before the kick. The ball ended up approximately 5.9 m from the left post. Bridging a gap of 3.15 m was too far from his position.
If Ronaldo was kicking the Jabulani instead, the ball would have moved a further 0.7 m towards De Gea ending up around 5.2 m from the left post, leaving De Gea to cover 2.45 m to stop the kick. The same kick with the Jabulani would probably have put the ball just within his reach. Accounting for the trigger motion towards the ball, that’s a kick he could have saved. Goalkeepers are beasts of instinct. If the ball was curving more towards him, instincts may have kicked in and he may have saved it (or atleast put in a valiant effort). Bye bye, Portugal!
Truth is the balls matter as much as the players. The difference between Portugal becoming champions and etching their name in history and Portugal going home; the difference between Ronaldo’s signature World Cup moment and a failure – history could have looked different with a different ball.
You see only minor differences in the wake for the Jabulani and the Telstar. The seam depth of Jabulani is 0.5 mm while that of the Telstar 18 is 1.1 mm. Miniscule? Yes. Negligible? No. It’s precisely the seam, in addition with the surface texture that makes different balls move differently for the same kick. The Jabulani had shallower seams with a smaller total seam length and a smoother surface. Hence, flow separates earlier resulting in a larger wake and a bigger deviation to the side.
What if it was a knuckleball?
Ronaldo, of course, is more famous for his knuckleball kicks, executed with no spin and at a high kick speed. The result is that the wake behind a non-spinning ball show more instability and larger fluctuations, leading to the knuckling effect. I was curious to see what would have happened Ronaldo had delivered a knuckleball instead. With some last minute computing power at my disposal, I was able to do so to analyze knuckleball aerodynamics.
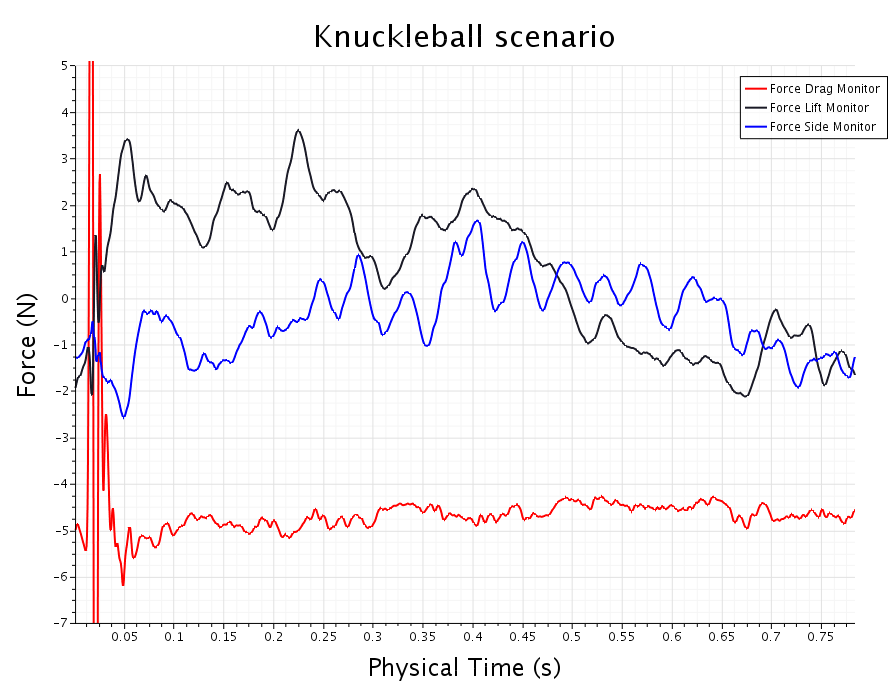
Knuckle ball aerodynamics for the Ronaldo free kick
Ronaldo would have had to hit the ball straight and up over the wall in this scenario instead of curving it from outside. Notice the side force changes direction throughout the flight – this is the knuckling effect. The lift on the ball also changes direction midway through the flight causing the unpredictable behavior. Though the average deviation isn’t much, it’s the change in direction to either side that makes it harder to stop. So why did Ronaldo ditch his trademark knuckleball? Because the distance to goal was too small to get the elevation and dip needed to go over the wall and into the goal.
Lift force – 0.58 N; Drag force – 4.65 N; Side force – 0.41 N.
Deviation towards the side – 0.59 N.
Conclusion
Whether Portugal wins it all or not, Ronaldo’s free kick will live on in the annals of the sport as one of its signature moments. Plenty of attention and praise has been heaped on him, all with good reason. The makers of the ball, Adidas, should also be commended for continuously pushing ball design and delivering a ball with ‘stable’ aerodynamics. These simulations are an attempt to further explain the physics behind the beautiful free kicks in football. Ronaldo kicks the ball away from the goal safe in the knowledge that the spin he imparted on the ball combined with the ball’s predictable behavior would be enough to swing it back and down towards the goal and still beat the keeper.
Would the kick have worked with the Jabulani? Probably not considering it would have brought the ball within the goalkeeper’s reach. Ball behavior can dictate the style of play as well. There’s a reason some teams relied solely on short passes (looking at you, Spain) in the 2010 World Cup just so they can counter unpredictable ball behavior in long passes.
As you watch the games, just remember that none of these goals ‘defy the laws of physics’ as the commentators like to call them. The difference between creating sports history and being a footnote in the world cup can often be explained by physics – ball design, those pesky turbulent vortices behind the ball and the boundary layer.
Now can someone get a hold of Cristiano? We would like him to execute the same kick with different world cup balls.
If you are interested in learning more about free kick physics, do check out the research of NASA’s Rabi Mehta and Lynchburg College’s John Eric Goff. Meanwhile, my colleagues from the system simulation branch of Simcenter are bringing out a new Simcenter Amesim World Cup library on July 5.
Adidas, Telstar 18 and Jabulani are registered trademarks of Adidas AG. Siemens PLM Software and its subsidiaries and products are not affiliated with, endorsed by, sponsored by, or supported by Adidas AG.
FIFA World Cup, 20148 FIFA World Cup, and 2010 FIFA World Cup are trademarks of FIFA. Siemens PLM Software and its subsidiaries and products are not affiliated with, endorsed by, sponsored by, or supported by FIFA.
Video footage courtesy of FOX Soccer